RBSE Solutions for Class 11 Physics Chapter 7 Rigid Body Dynamics

Rajasthan Board RBSE Class 11 Physics Chapter 7 Rigid Body Dynamics

RBSE Class 11 Physics Chapter 7 Textbook Exercises with Solutions

RBSE Class 11 Physics Chapter 7 Very Short Answer Type Questions

Question 1.
What is a rigid body?
Answer:
If under influence of external forces, there is no change in the shape of a body i.e., the molecular distance does not change it is known as a rigid body.

Question 2.
Define center of mass.
Answer:
The specific point of the body where all the mass of the body is supposed to be contained and the body moves such that all the external force act at this point, is known as the center of mass of the body.

Question 3.
Can the center of mass of a body be outside?
Answer:
Yes; for example the center of mass of a ring is at its center. Which is outside.

Question 4.
Write the relation between linear velocity and angular velocity.
Answer:
Relation between linear velocity (v) and angular velocity (ω) is,
v = rω
where, r is the radius of the circular path.

Question 5.
What is the angular speed of wrist watch’s minute needle in rad/s?
Answer:
∵ Angular velocity
ω = \(\frac{2 \pi}{T}\) rad/s
and the time perod of a minute needle;
1 hour = 3600 s.
∴ ω = \(\frac{2 \pi}{3600}\) rad/s
or ω = \(\frac{\pi}{1800}\) rad/s

Question 6.
What do you mean by the moment of inertia?
Answer:
The rotational inertness of a body in rotational motion is known as the moment of inertia of the body and it is measured by the product of mass of the body and the square of the distance of the body from the axis of rotation.

Question 7.
On what factors does the moment of inertia of an object depend?
Answer:
Moment of inertia of an object depends upon the following factors :

• On the position of the axis of rotation,
• On the mass of the object,
• On the distance of the mass from the axis of rotation.

Question 8.
How much is the moment of inertia of a ring along its diameter? ’
Answer:
Moment of inertia of a ring about its diameter.
I_d = \(\frac{1}{2}\) MR²
where, M is the mass of the ring and R is its radius.

Question 9.
The moment of inertia of a disc is minimum around which axis?
Answer:
Moment of inertia of the disc is minimum about its diameter and its value is:
I_d = \(\frac{1}{4}\) MR²
where, M is the mass and R the radius of the disc.

Question 10.
Write the value of radius of gyration of a solid sphere relative to its tangent.
Answer:
Moment of inertia of a solid sphere about its tangent, if radius of gyration be K, then
I = MK²
∴ MK² = \(\frac{7}{5}\) MR² ⇒K = \(R \sqrt{\frac{7}{5}}\)

Question 11.
Write the unit of angular momentum.
Answer:
∵ Angular momentum, J = I.ω
∴ Unit of J = kg.m²/s or J.s

Question 12.
A man is sitting on a moving table stretching his arms, if he brings his arms closer, what would be its effect on the moment of inertia?
Answer:
On closing the arms, the moment of inertia of the man will reduce.

Question 13.
If the rate of change of angular momentum is zero then what would be the value of torque acting on the body?
Answer:
∵ τ = \(\frac{\Delta J}{\Delta t}\) Here \(\frac{\Delta J}{\Delta t}\) = 0
∴ τ = 0

Question 14.
If an object is rotating then is it necessary that some torque is acting on it?
Answer:
Not necessary; because for uniform rotational motion (i.e, angular acceleration α = 0) any torque is not necessary. Torque is necessary to produce angular acceleration.

Question 15.
Write the relation between torque, moment of inertia and angular acceleration.
Answer:
Relation between torque (τ), moment of inertia (I) and angular acceleration (α) is.
τ = Iα

Question 16.
Why is the handle of screw driver large in width?
Answer:
The torque couple of forces, τ = F × r
where F is the magnitude of forces of couple and r is the distance between the forces (here the width of the handle of screwdriver). Therefore, to obtain much value of τ with small forces F, r is increased.

Question 17.
Write the value of total kinetic energy if an object is rotating along its axis and also moving in a straight line.
Answer:
E_t = \(\frac{1}{2} M v^{2}\left(1+\frac{K^{2}}{R^{2}}\right)\)
and this relation is obtained from the relation
E_t = \(\frac{1}{2}\) Mv² + \(\frac{1}{2}\) Iω²

Question 18.
Write the formula of velocity of a body rolling down on an inclined plane.
Answer:
v = \(\sqrt{\frac{2 g h}{\left(1+\frac{K^{2}}{R^{2}}\right)}}\), where h is the height of the inclined plane, R the radius of the body and K is the radius of gyration.

Question 19.
Write the conditions for mechanical equilibrium of rigid bodies.
Answer:
Conditions for mechanical equilibrium should satisfy it for rotational as well as linear motions of the bodies. These conditions are.
ΣF = 0 for linear motion
and Στ = 0 for rotational motion.

Question 20.
Write down the relation between angular momentum, moment of inertia and angular velocity.
Answer:
J = Iω; where J is angular momentum, I, the moment of inertia and ω, the angular velocity.

Question 21.
If the diameter of the earth reduces and becomes half then what would be the duration of a day?
Answer:
6 hours.

RBSE Class 11 Physics Chapter 7 Short Answer Type Questions

Question 1.
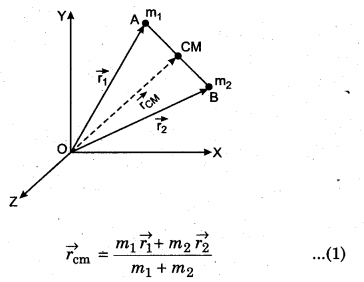
Establish relation between mass and distance of center of mass for a two particles system.
Answer:
Suppose, there are two particles in a system. Their masses are m₁ and m₂ respectively and their position vectors are \(\vec{r}_{1}\) and \(\vec{r}_{2}\) respectively. If the position vector of the center of mass of this system be \(\vec{r}_{\mathrm{cm}}\), then.

Proof: Suppose, external forces acting on these particle are \(\vec{F}_{1 \mathrm{ext}}\) and \(\vec{F}_{2\mathrm{ext}}\) respectively and internal forces are \(\vec{F}_{12}\) and \(\vec{F}_{21}\) respectively. Therefore, net force acting on particle no. 1,

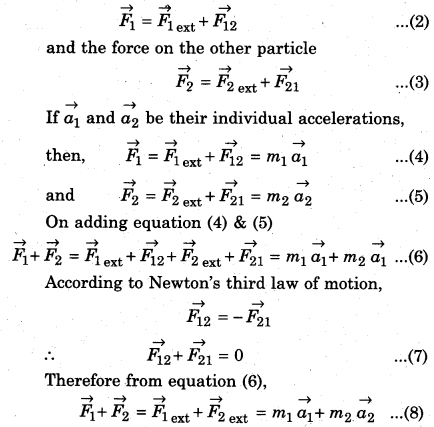
Suppose, at any moment the position vector, velocity vector and acceleration of center of mass are, \(\vec{r}_{\mathrm{cm}}, \vec{v}_{\mathrm{cm}} \text { and } \vec{a}_{\mathrm{cm}}\) respectively, then

Total mass of the system M = (m₁ + m₂). Since, according to concept of center of mass, all external forces act at the center of mass. Therefore, according to Newton’s second law of motion,

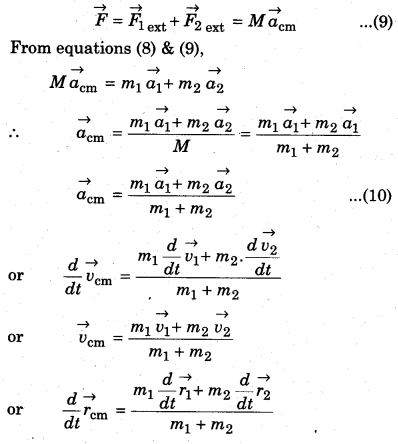
or \(\overrightarrow{r_{\mathrm{cm}}}=\frac{m_{1} \overrightarrow{r_{1}}+m_{2} \overrightarrow{r_{2}}}{m_{1}+m_{2}}\)

Question 2.
Establish vector relation between linear velocity and angular velocity.
Answer:
Vector relation between linear velocity and angular velocity :
When a rigid body rotates on its stable axis, then every particle of the body moves in a circular path. Every circle is situated on the rotational plane which is vertical to rotational axis and is centered on the axis. In pure rotational motion every particle of the rigid body moves with the same angular velocity. The value of the speed of the particle is directly proportional to its angular velocity. In the diagram, a rigid body particle P is moving in a circular path of radius r. If at any instant its angular displacement is ‘θ’ radian, then

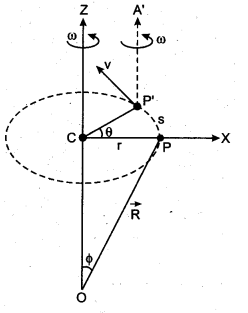
θ = \(\frac{\mathrm{arc}}{\text { radius }}=\frac{s}{r}\) ⇒ s = rθ
Differentiating the above equation with respect to time taking r constant, we get \(\frac{d s}{d t}=r \frac{d \theta}{d t}\)
Taking absolute value at both the ends;

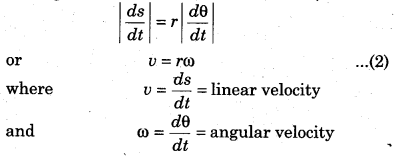
From equation(2), it is clear that the particle that is away from the axis of rotation, will move with more linear velocity.

To represent the above relationship in vector form, assume that the particle P has position vector \(\vec{R}=\overrightarrow{O P}\) at time t = 0. Therefore, from the figure,
\(\frac{r}{R}\) = sinϕ
From equation (2)
o = ωRsinϕ ………….. (3)
or \(\vec{v}=\vec{\omega} \times \vec{R}\) ……… (4)
This, is the vector relation between linear velocity and angular velocity.

Question 3.
Write the three equations of rotational motion.
Answer:
Equations of rotational motion,
(i) ω = ω₀ + αt
(ii) θ = ω₀t + \(\frac{1}{2}\)αt²
(iii) ω² = ω₀² + 2αθ
where: ω₀ = initial angular velocity,
ω = angular velocity at time t, α = angular acceleration
θ = angular displacement in time t.

Question 4.
Give the statement for theorem of perpendicular axis of moment of inertia.
Answer:
According to this theorem; “the moment of inertia of plane lamina about an axis perpendicular to its plane is the sum of the moments of inertia of two perpendicular axis lying in the plane of lamina and meeting at the scheduled axis.” i. e.,
I_Z = I_X + I_Y

Question 5.
Write the theorem of parallel axis of moment of inertia.
Answer:
According to this theorem, “the moment of inertia of a body about a rotational axis is equal to the moment of inertia relative to parallel axis passing through the center of mass plus the product of mass and square of the perpendicular distance between the parallel axis.” i.e,
I = I_CM + Mh²
where : I_CM = moment of inertia about an axis passing through center of mass
M = mass of the body
h = distance between the parallel axis.

Question 6.
Calculate the moment of inertia of a ring along an axis passing through the center and perpendicular to the plane.
Answer:
Moment of inertia of a ring about an axis passing through its center and perpendicular to its plane : Suppose a ring of mass M and radius R is placed in X- Y plane with its center at the origin of cartesian system. Z- axis will be our required axis in the question.

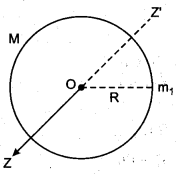
The mass of the ring is uniformly distributed to its whole circumference. Therefore, its whole circumference can be supposed to be made up of n
parts of masses respectively m₁, m₂, m₃, …………. , m_n.
Each mass element is situated at the same distance R from the Z-axis (i.e, schedued axis). Therefore the moment inertia of the ring,
I = I₁ + I₂ + I₃ + ……. +I_n
= m₁R² + m₂R² + m₃R² + …………… + m_nR²
= (m₁ + m₂ + m₃ + ……….. + m_n )R²
or I = MR²
where M = mass of ring.

Question 7.
Calculate the moment of inertia of a solid cylinder about its axis.
Answer:
Moment of inertia of solid cylinder about its axis : In the given diagram a cylinder (solid) of mass M, radius R and length l is shown. Suppose ZZ’ axis is its geometrical axis about which we have to calculate the moment of inertia. For the purpose, the whole length of the cylinder may be supposed to be made of many dish elements of vairous masses. Such type of one Therefore, moment of inertia of the whole cylinder,element is shown in the figure. Moment of inertia of this dish element about ZZ’ axis.

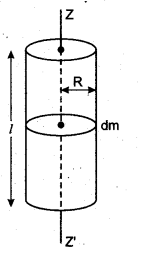
dI = \(\frac{1}{2}\)dmR²
Therefore, moment of inertia of the whole cylinder
I = ΣdI = Σ \(\frac{1}{2}\)dmR²
or I = \(\frac{1}{2}\)R²Σdm
or I = \(\frac{1}{2}\)MR²
where M = mass of the cylinder.

Question 8.
Establish a relation between torque and moment of inertia for a body.
Answer:
Relation between Torque, Moment of Inertia and Angular Acceleration
Let us consider a body on which torque x is applied, is rotating about an axis passing through a constant point and the body also has a constant angular acceleration α. All the particles of the body will have same angular acceleration a but the linear accelerations will be different. Suppose, one particle of the body has mass m₁ and the distance of it from the rotational axis is r₁. Then the linear acceleration of this particle is;
α₁ = r₁α
If \(\vec{F}\) is the force acting on the particle then;
F₁ = mass × acceleration
= \(m_{1} \vec{a}\) = m₁r₁α
The torque of this force along the rotational axis passing through O from the fig. 7.36 is;
τ₁ = Force (F₁) × distance (r₁)
= m₁r₁α × r = m₁r₁²α
Similarly, if m₂, m₃,… be the masses of other particles and r₂, r₃, … the distances from the rotational axis, then the torque acting on them will be; ‘c2 = m2r2a
τ₂ = m₂r₂²α
τ₃ = m₃ r₃²α
Since, the directions of all the torque is along the same rotational axis. Hence the resultant force (torque) on the body will be the vector sum of all the torques.
τ = τ₁ + τ₂ + τ₁ + …
= m₁r₁²α + m₂r₂²α + m₃r₃²α+ ……..
= (m₁r₁² + m₂r₂² + m₃r₃²) α
τ = (Σ mr²) α
But Σ mr² is the moment of inertia I about the rotational axis. Therefore,
τ = Iα ………….. (1)
Torque = Moment of inertia × Angular Acceleration
Keeping α = 1 rad/s² in the above equation,
τ = I
Hence, the moment of inertia of a body about the rotational axis is equal to the applied torque required to generate unit angular acceleration in the body.

Question 9.
Write the law of conservation of angular momentum. Write two examples based on this.
Answer:
Law of Conservation of Angular Momentum
The law of conservation of angular momentum states that “When the net external torque acting on a system about a given axis is zero, the total angular momentum of the system about that axis remains conserved.”
Mathematically, If Σ \(\vec{\tau}\) = 0 then \(\vec{J}\)= constant
Proof : According to the second law of motion, net force acting on a body is equal to its change of linear momentum i. e., \(\vec{F}=\frac{d \vec{P}}{d t}\)
Taking vector product of \(\vec{r}\) on both the sides of above expression
\(\vec{r} \times \vec{F}=\vec{r} \times \frac{\overrightarrow{d P}}{d t}\)
But \(\vec{r} \times \vec{F}\) is the torque acting on the body
∴ \(\vec{\tau}=\vec{r} \times \frac{\overrightarrow{d P}}{d t}\) …………….. (1)
Now angular momentum is defined as
\(\vec{J}=\vec{r} \times \vec{P}\)
Differentiating both the sides with respect to time (t)’

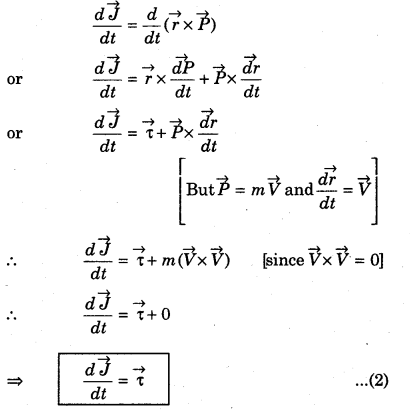
Which is the required equation.
This expression states that the torque acting on a particle is the time rate of change of its angular momentum. If the net external torque on the particle is zero, then,
\(\frac{d \overline{J}}{d t}\) = 0 or \(d \vec{J}\) = 0
Integrating both the sides
\(\vec{J}\) = constant
Thus the angular momentum of a particle is conserved if the net external torque acting on the particle is zero.
\(\vec{J}\) = Iω = constant
or I ∝ \(\frac{1}{\omega}\)
A system may consist of many number of particles or bodies. In case, there is a single non-rigid body, then during rotation, its moment of inertia may vary due to the change of distribution of mass about the axis of rotation. Therefore, in such a case (a single non rigid body), if moment of inertia changes from I₁ to I₂, then angular velocity must change from ω₁ to ω₂, so that
I₁ω₁ = I₂ω₂
Applications of Law of Conservation of Angular Momentum
Following are examples of some observed physical phenomena, which can be explained on the basis of the law of conservation of angular momentum :
(1) The angular velocity of a planet revolving in an elliptical orbit around the sun increases, when it comes near the sun and vice-versa : When the planet moving along its elliptical orbit is near the sun, its moment of inertia about the axis through the sun decreases and therefore its angular speed increases. On the other hand, when it is far away from the sun, its moment of inertia increases and hence angular speed decreases.

(2) A circus acrobat performs feats involving spin by bringing his arms and legs closer to his body or vice-versa. On bringing the arms and legs closer to the body, his moment of inertia / decreases. Hence to increases.

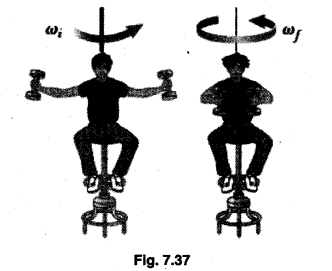
Question 10.
Calculate the kinetic energy of a body, rotating about an axis with uniform angular velocity.
Answer:
Rotational Kinetic Energy
Suppose a body is rotating with uniform angular velocity co relative to the axis YY’. Every particle of the body will have same angular velocity but different linear velocity. Suppose, a particle of mass m₁ is at a distance r₁ from the rotational axis. Since, the linear velocity of each particle is the multiplication of the angular velocity with the distance from the rotational axis. Hence, linear velocity of the particle.
v₁ = r₁ω
and its kinetic energy

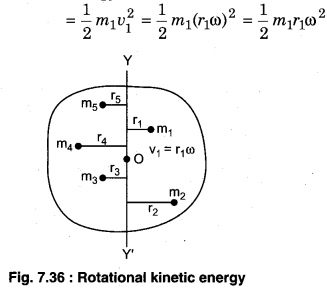
Similarly, if the masses of other particles are m₂, m₃,… and distances from the rotational axis are r₂, r₃,… then their kinetic energy will be \(\frac{1}{2}\) m₂r₂²ω², \(\frac{1}{2}\) m₃r₃²ω², … respectively since, kinetic energy is a scalar quantity, hence the total kinetic energy will be the sum of kinetic energies of all the particles.
E_R = \(\frac{1}{2}\) m₁r₁²ω² + \(\frac{1}{2}\) m₂r₂²ω² + \(\frac{1}{2}\) m₃r₃²ω² + ………
= \(\frac{1}{2}\) (m₁r₁² + m₂r₂² + m₃r₃² + …………) ω²
= \(\frac{1}{2}\) (Σ mr²) ω²
But, Σ mr² , is the moment of inertia I of the figid body about the axis
Therefore; E_R = \(\frac{1}{2}\) Iω² …………… (1)
This is the formula for kinetic energy of uniform rotation. It is clear from this that the linear kinetic energy \(\left[\frac{1}{2} m v^{2}\right]\) of a body is half the multiplication of mass m and linear velocity v². Similarly, the rotational kinetic energy \(\left[\frac{1}{2} I \omega^{2}\right]\) is half the multiplication of the moment of inertia I and angular velocity ω².
Keeping ω = 1 in equation (1) ;
I = 2E
Hence, if a body is moving with unit angular velocity along an axis then the moment of inertia of the body along the axis would be double its rotational kinetic energy. In other words rotational kinetic energy would be half of moment of inertia.
If any body is moving along its axis and also performing linear motion (e.g., moving motor or cycle’s wheel); then its total kinetic energy will be equal to the sum of rotational kinetic energy and linear kinetic energy.
E_total = \(\frac{1}{2}\) Iω² + \(\frac{1}{2}\) Mv² ………….. (2)

Question 11.
Establish the formula for acceleration of rolling body on an inclined plane.
Answer:
Rolling Motion on an Inclined Plane
It is an example of the rigid body motion, in which the centre of mass is also in motion.

Consider a solid object (sphere) of radius R and mass m rolling down a plane inclined at an angle 0 to the horizontal (figure 7.39).The height of the inclined plane is h and the distance is s. If the object rolls down from the highest point of the inclined plane then at that time the total energy at point C = potential energy
= mgh …………. (1)
In this motion the object does the rotational motion about an axis passing through the center of mass as well as the translatory motion along the plane.

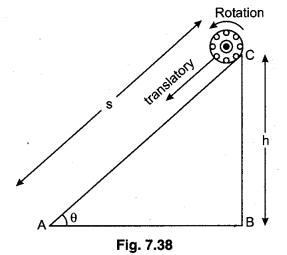
Thus, Rolling motion = Translatory motion + Rotatory motion.
At the bottom of the plane A, its velocity becomes v and angular velocity ω, then
Total energy at point A= Translatory kinetic energy + rotational energy
= \(\frac{1}{2}\) mv² + \(\frac{1}{2}\) Iω²
Hence, by the law of conservation momentum


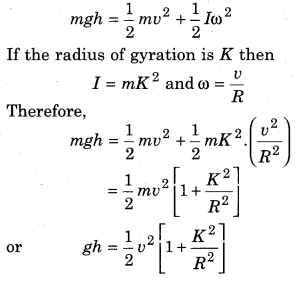
This is the representation of the velocity of a body at an inclined plane.
If the initial velocity of the body is zero (u = 0) then by the equation of motion (III ^rd equation)

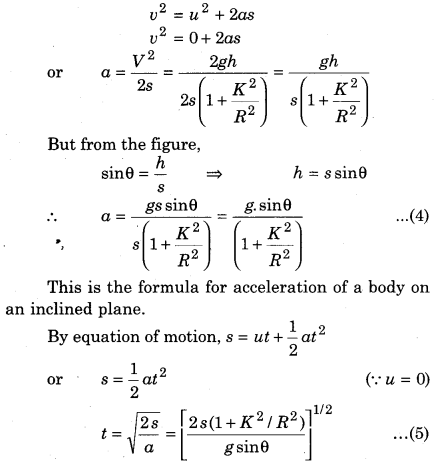
If the objects of various shapes are rolled down an inclined plane then the object whose K² / R² is minimum, its value of velocity and acceleration will be maximum when it reaches the end; and it will reach first at the bottom; the object whose K² / R² value is maximum will take maximum time to reach the end.

The value of velocity, acceleration and time are not depend upon the mass of a rolling body on an inclined plane. For similar value of h, the value of velocity is not depend upon the angle ‘θ’ of the inclined plane.

The value of K² / R² for main objects rolling down an inclined plane are as follows :
For solid sphere,
I = MK² = \(\frac{2}{5}\) MR² ⇒ \(\frac{K^{2}}{R^{2}}=\frac{2}{5}\)

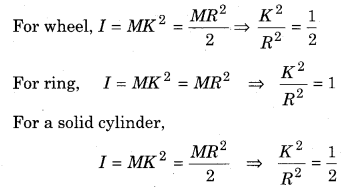
If all the objects are dropped down together on an inclined plane, then sphere will reach first and ring will be last. Wheel and solid cylinder will reach together at the end.

Question 12.
What is the physical importance of moment of inertia?
Answer:
Physical importance of moment of inertia : The moment of inertia is very important in our daily life. To increase the moment inertia of the wheels of scooter, motor cycle, bicycle, riksha, tanga and bull cart etc. most of the material i.e., is on the circumference which is connected by spokes with the axis. By riding on a bicycle and then leaving the pedal it can move for some time i. e., upto some distance due to more moment of inertia, which means the wheels keeps on moving.

The use of moment of inertia is very significant in automobile areas. Automobile engines which generate rotational energy, has a flywheel which is a wheel with high moment of inertia. When the value of torque increases or decreases which rotates the shaft, then the flywheel due to very high moment of inertia moves with a constant speed, due to which we are saved from high shocks.

The motor toys of children also have flywheel. It is rubbed on the ground and then left due to which it moves upto some distance.

Question 13.
Calculate the moment of inertia of a thin rod about an axis perpendicular to the length and passing through one edge.
Answer:
Moment of Inertia of a Thin Rod
(a) Moment of Inertia of a Thin Rod about the Axis Perpendicular to the Length and Passing through the Center
Take a uniform thin rod AB whose mass is M and length is l. YY’ axis passes through the center of the rod and perpendicular to the length of the rod. We have to calculate the moment of inertia about this axis. YY’.

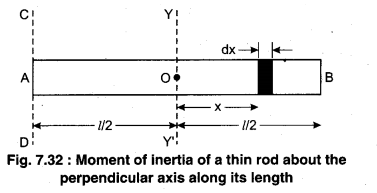
Suppose, small piece dx is at a distance x from the YY’ axis. Hence, mass of length dx is = \(\left(\frac{M}{l}\right)\) dx
The moment of inertia of small piece about the YY’ axis
dl = \(\left[\left(\frac{M}{l}\right) d x\right] x^{2}\)
Therefore, the moment of inertia of the complete rod about the YY’ axis.

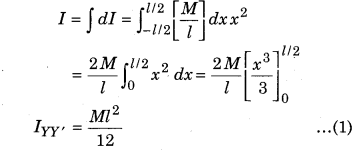
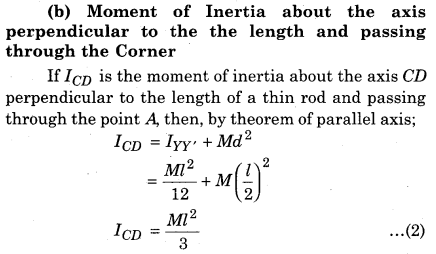
(b) Moment of Inertia about the axis perpendicular to the the length and passing through the Corner
If I_CD is the moment of inertia about the axis CD perpendicular to the length of a thin rod and passing through the point A then, by theorem of parallel axis;
I_CD = I_YY’ + Md²
= \(\frac{M l^{2}}{12}+M\left(\frac{l}{2}\right)^{2}\)
I_CD = \(\frac{M l^{2}}{3}\) ……………….. (2)

Question 14.
Derive the formula of total kinetic energy of a body at the bottom rolling down on an inclined plane.
Answer:
Total kinetic energy of a body at the bottom rolling down on an inclined plane E = Translational kinetic energy + Rotational kinetic energy

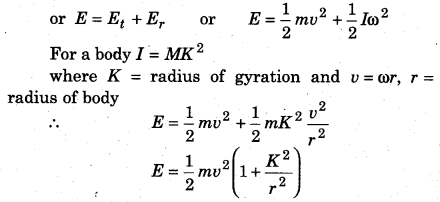
It is the formula of kinetic energy.

Question 15.
Establish the relation between angular momentum and angular velocity.
Answer:
Relation between Angular Momentum, Moment of Inertia and Angular Velocity
We know that the angular momentum of a body is given as;
\(\vec{J}=\vec{r} \times \vec{p}\)
or J = r p sinθ \(\hat{n}\)
If \(\vec{r}\) and \(\vec{p}\) are perpendicular, then θ = 90°
sin 90° = 1
J = r p
= r m v
= r m(r ω) (∵ v = rω)
J = mr²ω
The angular momentum of the body will be equal to the kum of all the moments of linear momentums of the particles i.e., angular momentum relative to the rotational axis
J = Σ mr² ω
∵ ω is constant, hence J = ω Σ mr²
J = Iω (I = Σ mr²) …………… (1)
If ω = 1 rad/s then I = J
Hence, the moment of inertia of a body about the axis is equal to the angular momentum if it is rotating with unit angular velocity.

RBSE Class 11 Physics Chapter 7 Long Answer Type Questions

Question 1.
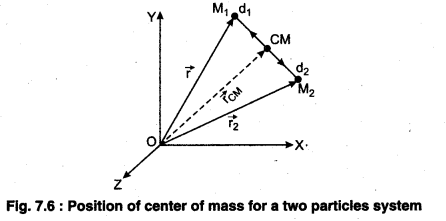
What do you mean by center of mass ? Calculate the position coordinates of the center of mass of two particles in a system.
Answer:
Center of Mass
Introduction of Center of Mass

• Untill now we have focused on describing motion of a single particle in one, two or three dimensions. By particle we mean to say that it has a size negligible in comparison to the path travelled by it.
• When we study laws of motion, we apply them’ even to the bodies having finite size imagining that motion of such bodies can be described in terms of motion of particles.
• While doing so we have ignored the internal structure of such bodies. Any real body we encounter in our daily life has a finite size and idealized model of particle is inadequate when we deal with motion of real bodies of finite size.
• Real bodies of finite size can also be regarded as the system of particles. While studying
  system of particles, we will not concentrate on each and every particle of the system, instead we will consider the motion of system as a whole.
• Large number of problems involving extended bodies or real bodies of finite size can be solved by considering them as rigid bodies. We define rigid body as a body having definite and unchanging shapes.
• A rigid body is rigid assembly of particles with fixed inter particle distances. In actual bodies deformation do occur but we neglect them for the sake of simplicity.

Center of Mass of Two Particle System
(i) Position of center of mass for two particle system : Consider a system of two point masses (or particles) M₁ and M₂, whose position vectors at time t with reference to the origin O of the inertial frame are \(\vec{r}_{1}\) and \(\vec{r}_{2}\) respectively as shown in the figure.
The total force \(\vec{F}_{1}\) that is acting on point mass M₁ consists of two parts :
(i) A force (\(\vec{F}_{1}\))_ext which appears because of some agency acting on the system.
(ii) A force \(\vec{F}_{12}\) which appears because of point mass M₂. This force is internal force of the two. particles system

So, total force is
\(\vec{F}_{1}=\vec{F}_{1} \cdot_{e x t}+\vec{F}_{12}\) ……………… (1)
Similarly the force on the other particle (for point mass M₂)

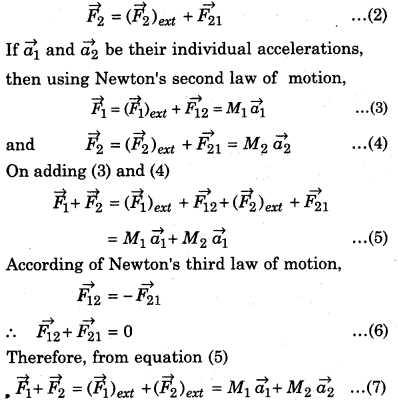
We know that all external forces act at the center of mass. If total mass of the system is
M = M₁ + M₂, then from equation (7)

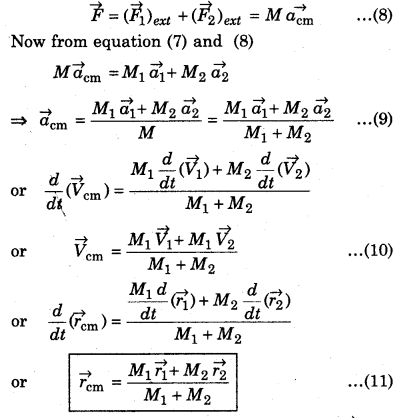
The point whose position is defined by \(\vec{r}_{\mathrm{cm}}\) is called the center of mass of a two particles system. So equation (11) gives the center of mass formula for a particle system at any time ‘t’. It is a point at which the total external force is supposed to be acting.

Question 2.
Give the statement of theorems of moment of inertia and prove them.
Answer:
Theorems of Moment of inertia
In the case of the rigid bodies having regular shapes, the axis through the center of mass is usually a symmetric axis. It will be seen that it is always convenient to find out moment of inertia of the body about the axis through its center of mass. However, moment of inertia about some other axis can be found with the help of theorems of parallel and perpendicular axis.

(a) Theorem of Perpendicular Axis : The perpendicular axis theorem (or plane figure theorem) can be used to determine the moment of inertia of a rigid body that lies entirely with in a plane.

This theorem states that the moment of inertia about an axis perpendicular to the plane is equal to the sum of the moments of inertia of two perpendicular axis through the same point in the plane of the object.

Explanation : Let OZ be the axis perpendicular to the plane lamina and passing through point ‘O’. Let OX and OY be two mutually perpendicular axis in the plane of lamina and intersecting at O’. It I_X, I_Y and I_Z are the moments of inertia of the plane lamina about the axis OX,OY and OZ respectively, then according to the theorem of perpendicular axis :
I_Z = I_X + I_Y
Proof : Let the lamina consists of n number particles,of masses m₁, m₂, m₃ …….. m_n. Their positions are at the distances r₁, r₂, r₃ ………. r_n respectively from the origin O. For simplicity, let a particle of mass m₁ is at point P(x₁, y₁) and its position is denoted by r₁

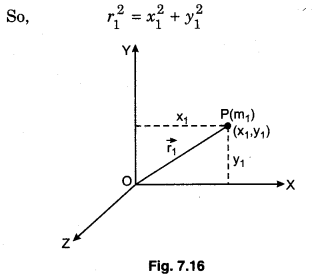
The moment of inertia of the particle of mass m₁, about OZ axis is :
I₁ = m₁r₁² = m₁ (x₁² + y₁²)
Similarly the inertia of the particle of mass m₂ about OZ axis is
I₂ = m₂ (x₂² + y₂²)
Therefore, the moment of inertia of the whole lamina about OZ axis is :
I_Z = I₁ + I₂ + I₃ + …………… I_n
= m₁ (x₁² + y₁²) + m₂ (x₂² + y₂²) + ………… + m_n (x_n² + y_n²)
or I_Z = (m ₁x₁² + m₂x₂² + m₃x₃² + …………. + m_nx_n²) + (m₁y₁² + m₂y₂² + m₃y₃² + …………. + m_ny_n²)
or I_Z = I_P + I_Y Proved

(b) Theorem of Parallel Axis : It states that the moment of inertia of rigid body about any axis is equal to its moment of inertia about a parallel axis through its center of mass plus the product of mass of the body and the square of the perpendicular distance between the two axis.

Let I_C be the moment of inertia of a body of mass M about an axis A’ B’ passing through its center of mass C. Let I be the moment of inertia of the body about an axis AB parallel to the axis A’ B’ and at a distance I? from it. [figure 7.17]

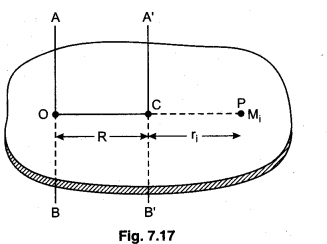
Then according to the theorem of parallel axis,
I = I_C + MR²
Proof : Consider that tth particle located at the point P in the body, is of the mass m_i and lies at a distance r_i from the axis A’B’. Then, the distance of the ith particle from the axis AB is (r_i + R).
The moment of inertia of the ith particle about the axis A’B’ is (m_ir_i²).
Therefore, moment of inertia of the body about the axis A’B’ is given by
I_C = \(\sum_{i=1}^{n} m_{i} r_{i}^{2}\) ………… (1)
Also, moment of inertia of the particle about the axis AB is m_i (r_i + R)², so that moment of inertia of the body about the axis AB is given by

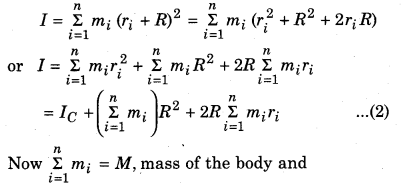
\(\sum_{i=1}^{n} m_{i} r_{i}\) = Sum of the moments of the masses of the particles constituting the body about the axis’ through its center of mass. Since, sum of the moment of the masses of the particles constituting the body
about an axis through its center of mass must be zero, n
∴ \(\sum_{i=1}^{n} m_{i} r_{i}=0\)
In equation (2) substituting the value of the two factors, we havei
I = I_C + MR² …………. (3)
It proves the theorem of parallel axis for moment of inertia.

Question 3.
Calculate the moment of inertia of a disc along the axis and perpendicular to the axis passing through the center.
Answer:
Moment of Inertia of a Circular Disc
Moment of Inertia of a Circular Disc about the Axis Perpendicular to the Plane and Passing Through the Center of the Disc
Diagram (7.22) shows a circular disc of radius R with center O. If M is the mass of the, disc then the mass of the unit area of the surface of the disc will be represented as (σ).

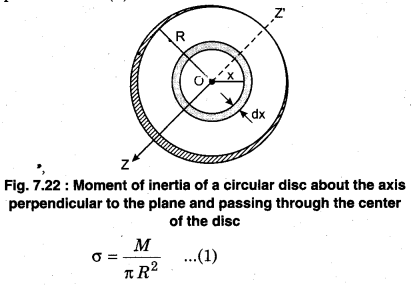
We can assume that the disc is made up of many same centered circular rings. Suppose that x is the radius of one of those circular ring; and its width is dx, then;
Area of the ring = circumference × width
= 2 π x dx
Mass of this ring ⇒ dM = 2 π x dxσ
All the particles of this ring are at the same distance from the center. Hence, the moment of inertia of the ring about the axis perpendicular to the plane and passing through the center is;
dl = mass of the ring × (radius)²
= 2 π x dxσ x²
= 2 π x³ dxσ …………… (2)
Now, moment of inertia of the total disc will be equal to the sum of moment of inertia of all the circular rings which are between x = 0 and x = R. Therefore;
I = \(\int d I=\int_{0}^{R} 2 \pi x^{3} \sigma d x\)
= \(2 \pi \sigma \int_{0}^{R} x^{3} d x\)

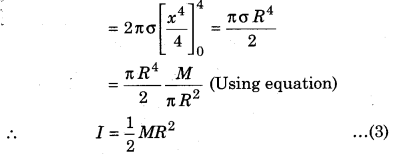
Question 4.
Calculate the moment of inertia of a solid cylinder along the axis passing through the center of mass and perpendicular to its length.
Answer:
Moment of Inertia of the Cylinder about the Axis Perpendicular to the Length of the Cylinder and Passing Through the Center
According to the figure (7.27) a cylinder of mass M, length l and radius R is shown; which is made up of coaxial discs of radius R. We have to calculate the moment of inertia about the YY’ axis perpendicular to XX’ and passing through the centre O. If there is a disc of width dx and at a distance x from the YY’ axis then;
Volume of the disc = Area × thickness = πR²dx
If ρ is the density of the cylinder, then ρ = \(\frac{M}{\pi R^{2} l}\)
dm = (Volume) × mass per unit volume of the cylinder
Therefore, mass of the disc (dm) = πR²dxρ

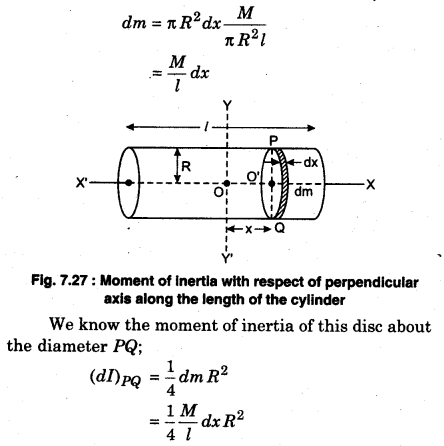
By the theorem of parallel axis, the moment of inertia of this disc about the YY’ axis.

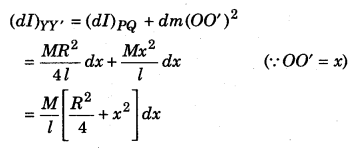
The moment of inertia of the whole cylinder about the YY’ axis will be equal to the sum of moment of inertia of all these discs which are between

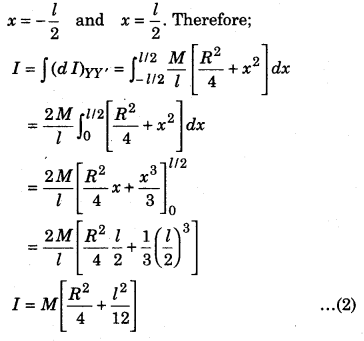
Question 5.
Calculate the moment of inertia of a solid sphere about its diameter.
Answer:
Moment of Inertia of a Solid Sphere about its Diameter
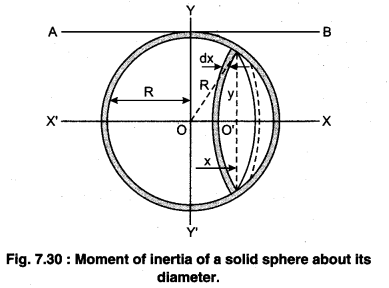
According to the figure (7.30) a sphere of mass M and radius R is shown, whose density is p. We have to calculate the moment of inertia of the sphere about the diameter XX’.

We can assume the sphere to be made up of many discs whose surfaces are parallel to YY’ and the center is on XX’ axis. One of these discs has a center at O’ and radius y; and the distance of the O’ circle from center O is x; the width of this disc is dx.

Density of the sphere (ρ) = \(\frac{M}{\frac{4}{2} \pi R^{3}}\) ……………. (1)
Volume of the disc = π y²dx
and the mass of the disc = π y²dx ρ …………….. (2)
Therefore, the moment of inertia of the sphere about the axis XX’ perpendicular to the surface (plane) and passing through the center is;

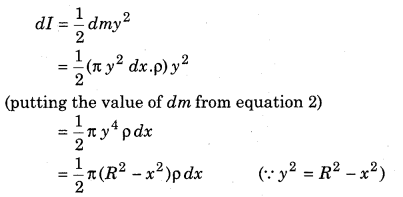
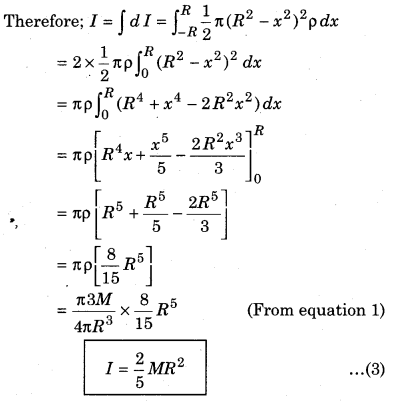
The moment of inertia of the total sphere about the XX’ axis will be equal to the sum of the moment of inertia of all the discs between x = -R and x = +R.

Question 6.
Establish the formula of moment of inertia of a rectangular rod along the axis passing through its center of mass and perpendicular to the length of the rod.
Answer:
Solid Rod of Rectangular Cross Section
Moment of Inertia about the axis perpendicular to the length and passing through the Center of Mass
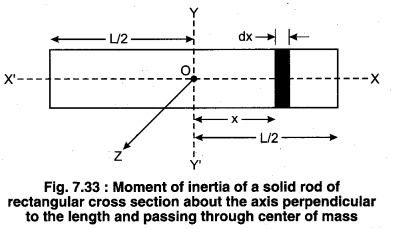
A rectangular solid rod is shown in the figure (7.33), whose length is L, breadth B, width d and mass is M. We can assume that this is made up of various thin rectangular cross-sections. The total moment of inertia of the rod would be equal to the sum of the moment of inertia of all these rectangular cross-sections.

Suppose every rectangular cross-section’s mass is m. According to the figure (7.33) a cross-section of dx breadth is at a distance x parallel to the YY’ axis. If mass of unit area of the strip is a then the whole cross-section mass is B dxσ. Therefore, the moment of inertia about the YY’ axis is = B dxσ x²

The moment of inertia of the total rectangular cross-section about the YY’ axis

Here, m = σBL is the mass rectangular cross-section.
Similarly, the moment of inertia about the XX’ axis is;
dI_XX’ = \(\frac{m B^{2}}{12}\)
dI_XX’ and dI_YY’ are the moment of inertia of two perpendicular axis in the same plane. Figure (7.33) shows the axis OZ perpendicular to the intersection point of these two axis and its inertia;
dI_OZ = dI_YY’ + dI_XX’
\(=\frac{m L^{2}}{12}+\frac{m B^{2}}{12}=m\left[\frac{L^{2}+B^{2}}{12}\right]\)
The moment of inertia of such rectangular laminas will be the sum of all about the Z axis.
Hence, the moment of inertia about the OZ axis, which is perpendicular to length and passing through the center is;

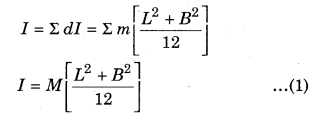
Question 7.
Calculate the moment of inertia of a hollow sphere along its diameter.
Answer:
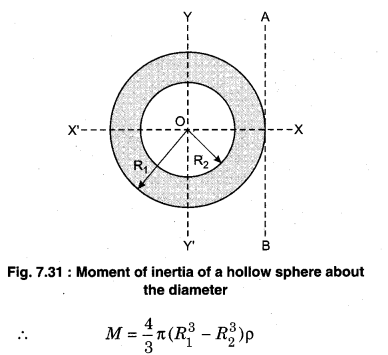
Moment of Inertia of a Hollow Sphere
Moment of Inertia of a Hollow Sphere about the Diameter
Suppose the mass of a hollow sphere is M, ρ is the density, inner radius R₂ and outer radius R₁, (diagram 7.31).

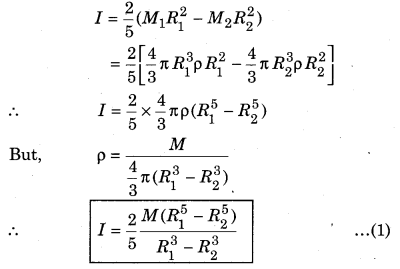
Moment of inertia of a hollow sphere (I)
= Moment of inertia of a solid sphere of radius R₁
– Moment of inertia of a solid sphere of radius R₂

Question 8.
Prove that the kinetic energy of a body in rotational motion is \(\frac{1}{2}\)Iω²
Answer:
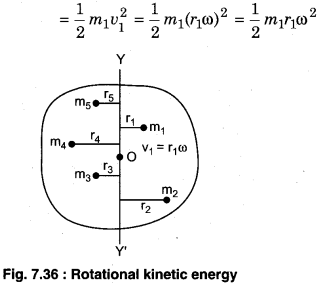
Rotational Kinetic Energy
Suppose a body is rotating with uniform angular velocity co relative to the axis YY’. Every particle of the body will have same angular velocity but different linear velocity. Suppose, a particle of mass m₁ is at a distance r₁ from the rotational axis. Since, the linear velocity of each particle is the multiplication of the angular velocity with the distance from the rotational axis. Hence, linear velocity of the particle.
v₁ = r₁ω
and its kinetic energy

Similarly, if the masses of other particles are m₂, m₃,… and distances from the rotational axis are r₂, r₃,… then their kinetic energy will be \(\frac{1}{2}\) m₂r₂²ω², \(\frac{1}{2}\) m₃r₃²ω², … respectively since, kinetic energy is a scalar quantity, hence the total kinetic energy will be the sum of kinetic energies of all the particles.
E_R = \(\frac{1}{2}\) m₁r₁²ω² + \(\frac{1}{2}\) m₂r₂²ω² + \(\frac{1}{2}\) m₃r₃²ω² + ………
= \(\frac{1}{2}\) (m₁r₁² + m₂r₂² + m₃r₃² + …………) ω²
= \(\frac{1}{2}\) (Σ mr²) ω²
But, Σ mr² , is the moment of inertia I of the figid body about the axis
Therefore; E_R = \(\frac{1}{2}\) Iω² …………… (1) This is the formula for kinetic energy of uniform rotation. It is clear from this that the linear kinetic energy \(\left[\frac{1}{2} m v^{2}\right]\) of a body is half the multiplication of mass m and linear velocity v². Similarly, the rotational kinetic energy \(\left[\frac{1}{2} I \omega^{2}\right]\) is half the multiplication of the moment of inertia I and angular velocity ω².
Keeping ω = 1 in equation (1) ;
I = 2E
Hence, if a body is moving with unit angular velocity along an axis then the moment of inertia of the body along the axis would be double its rotational kinetic energy. In other words rotational kinetic energy would be half of moment of inertia.
If any body is moving along its axis and also performing linear motion (e.g., moving motor or cycle’s wheel); then its total kinetic energy will be equal to the sum of rotational kinetic energy and linear kinetic energy.
E_total = \(\frac{1}{2}\) Iω² + \(\frac{1}{2}\) Mv² ………….. (2)

Question 9.
Calculate the moment of inertia of a symmetrical thin rod along the axis passing through its end and perpendicular to its length.
Answer:
Moment of Inertia of a Thin Rod
Moment of Inertia of a Thin Rod about the Axis Perpendicular to the Length and Passing through the Center
Take a uniform thin rod AB whose mass is M and length is l. YY’ axis passes through the center of the rod and perpendicular to the length of the rod. We have to calculate the moment of inertia about this axis. YY’.

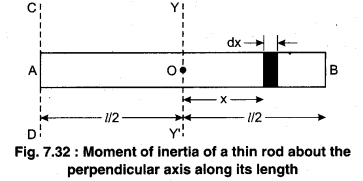
Suppose, small piece dx is at a distance x from the YY’ axis. Hence, mass of length dx is = \(\left(\frac{M}{l}\right)\) dx
The moment of inertia of small piece about the YY’ axis
dl = \(\left[\left(\frac{M}{l}\right) d x\right] x^{2}\)
Therefore, the moment of inertia of the complete rod about the YY’ axis.

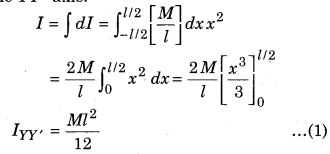
Question 10.
Establish the formula for velocity and acceleration of a rolling body on an inclined plane.
Answer:
Rolling Motion on an Inclined Plane
It is an example of the rigid body motion, in which the centre of mass is also in motion.

Consider a solid object (sphere) of radius R and mass m rolling down a plane inclined at an angle 0 to the horizontal (figure 7.39).The height of the inclined plane is h and the distance is s. If the object rolls down from the highest point of the inclined plane then at that time the total energy at point C = potential energy
= mgh …………. (1)
In this motion the object does the rotational motion about an axis passing through the center of mass as well as the translatory motion along the plane.

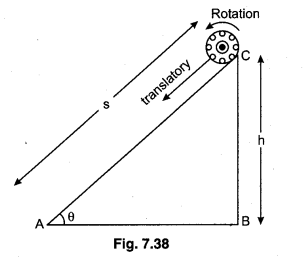
Thus, Rolling motion = Translatory motion + Rotatory motion.
At the bottom of the plane A, its velocity becomes v and angular velocity ω, then
Total energy at point A= Translatory kinetic energy + rotational energy
= \(\frac{1}{2}\) mv² + \(\frac{1}{2}\) Iω²
Hence, by the law of conservation momentum


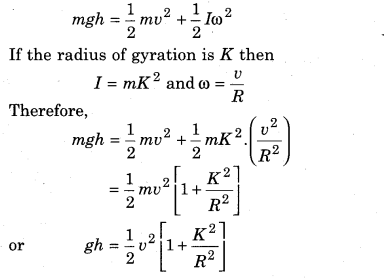
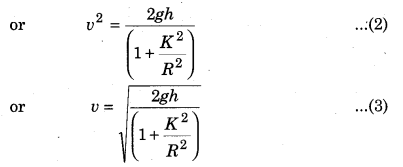
This is the representation of the velocity of a body at an inclined plane.
If the initial velocity of the body is zero (u = 0) then by the equation of motion (III ^rd equation)

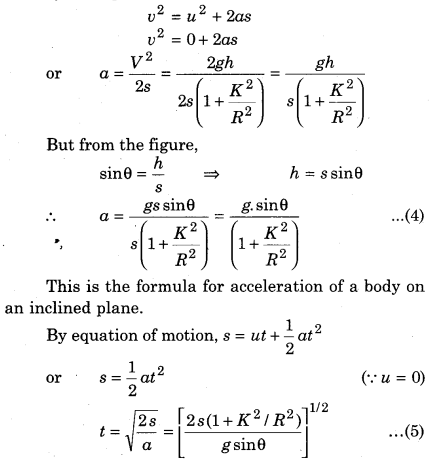
If the objects of various shapes are rolled down an inclined plane then the object whose K² / R² is minimum, its value of velocity and acceleration will be maximum when it reaches the end; and it will reach first at the bottom; the object whose K² / R² value is maximum will take maximum time to reach the end.

The value of velocity, acceleration and time are not depend upon the mass of a rolling body on an inclined plane. For similar value of h, the value of velocity is not depend upon the angle ‘θ’ of the inclined plane.

The value of K² / R² for main objects rolling down an inclined plane are as follows :
For solid sphere,
I = MK² = \(\frac{2}{5}\) MR² ⇒ \(\frac{K^{2}}{R^{2}}=\frac{2}{5}\)

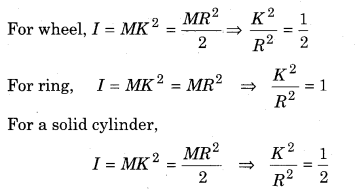
If all the objects are dropped down together on an inclined plane, then sphere will reach first and ring will be last. Wheel and solid cylinder will reach together at the end.

RBSE Class 11 Physics Chapter 7 Numerical Questions

Question 1.
Two particles of masses 5 g and 2 g respectively; have velocity \((\hat{i}+\hat{j}-\hat{k})\) and \((3 \hat{i}-2 \hat{j}+\hat{k})\) cm/s; and form a two particles system. What will be the velocity of the center of mass of the system?
Solution:
Given; m₁ = 5g; m₂ = 2g;
\(\overrightarrow{v_{1}}=(\hat{i}+\hat{j}-\hat{k})\) cm/s and \(\overrightarrow{v_{2}}=(3 \hat{i}-2 \hat{j}+\hat{k})\); \(\vec{v}_{\mathrm{cm}}\) =?
The velocity of center of mass,
\(\vec{v}_{\mathrm{cm}}=\frac{1}{M}\left(m_{1} \overrightarrow{v_{1}}+m_{2} \overrightarrow{v_{2}}\right)\)

Question 2.
A wheel which is at rest, rotates with 3.0 rad/s² angular acceleration for 2.0 s. What will be the angular velocity gained and how much would be the displacement in this time interval?
Solution:
Given; ω₀ = 0; α = 3.0 rad/s²; t = 2.0 s;
ω = ?; θ = ?
From first equation of motion,
ω = ω₀ + αt = 0 + 3.0 × 2
or ω = 6.0 rad/s
Now applying second equation,
θ = ω₀t + \(\frac{1}{2}\) αt² = 0 + \(\frac{1}{2}\) × 3 × 2 × 2
or θ = 6.0 rad

Question 3.
A car which is at rest is accelerated with an acceleration of 40 rad/s². In how much time will it get the angular velocity of 800 cycles/minute?
Solution:
Given; ω₀ = 0; α = 40 rad/s; t = ?

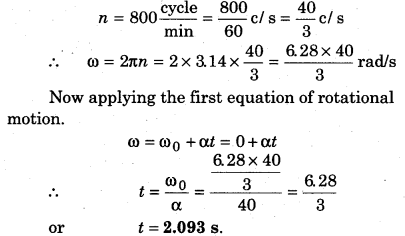
Question 4.
Calculate the center of mass of three particles kept on the vertices of an equilateral triangle. The masses of the particles are 100 g, 150 g and 200 g. The length of each side of the triangle is 0.5 m.

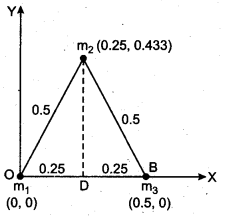
Solution:
Given; m₁ = 100 g; m₂ = 150 g; m₃ = 200 g,
l = 0.5 m, x_cm =?, y_cm = ?
From Pythagoras theorem,
AD² = OA² – OD²
= (0.5)² – (0.25)² = 0.25 – 0.0625
or AD² = 0.1875
∴ AD = \(\sqrt{0.185}\) = 0.433m
or AD = 0.433 m
Therefore, the co-ordinates of point O, x₁ = 0, y₁ = 0, coordinates of point B are x₂ = 0.5 m, y₂ = 0
and co-ordinates of points C are x₃ = 0.25 m, y₃ = 0.433 m.
If x_cm and y_cm be the co-ordinates of center of mass then,

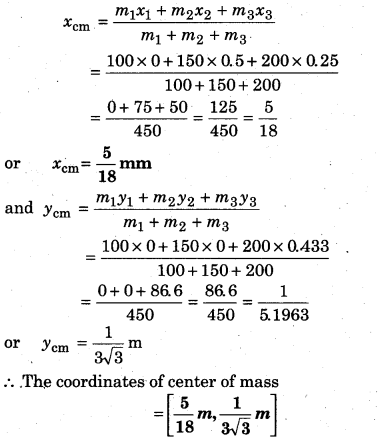
Question 5.
To generate angular acceleration of 4 rad/s² in a rotating body a torque of 2.0 × 10^-4 Nm is applied. What is the moment of inertia of the body?
Solution:
Given; α = 4 rad/s²; T = 2.0 × 10^-4 Nm; I = 1, The relation between τ, I and α is,
τ = Iα
∴ \(I=\frac{\tau}{\alpha}=\frac{2.0 \times 10^{-4}}{4}=\frac{1}{4} \times 10^{-4}=0.5 \times 10^{-4}\)
or I = 5.0 × 10^-5 kgm²

Question 6.
The two atomic molecules have masses m₁ and m₂ in a diatomic molecule. The distance between them is a m. Calculate the moment of inertia of the system along the axis passing through the center of gravity and perpendicular to the line joining the molecules.
Solution:
The arrangement of atoms in a diatomic molecule is shown in the adjoining figure. In a body or system of particles having regular size and shape, the center of gravity and the center of mass, coincide, and the sum of moment of masses about C.G. is zero.


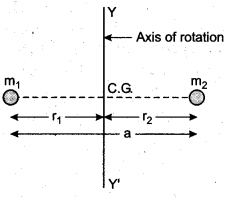
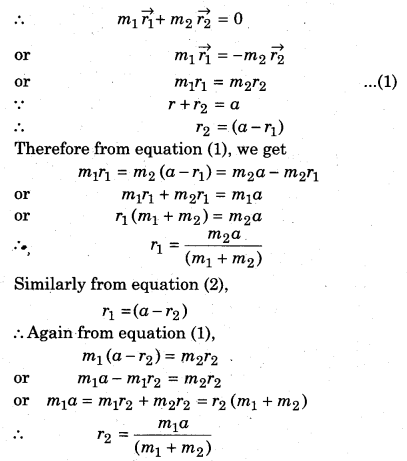
Therefore, moment of inertia of the molecule about the axis YY’

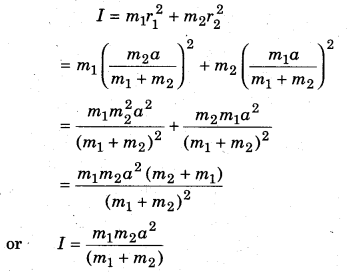
Question 7.
A circular disc whose radius is 0.5 m and mass 25 kg is rotating on its axis with 120 revolutions/minute. Calculate the moment of inertia of the disc and rotational kinetic energy.
Solution:
Given Radius of the disc, R = 0.5 m;
Mass of the disc, M = 25kg; / = ?; E_r = ?;
Frequency = 120 rev/min = \(\frac{120}{60}\) rev/s = 2 Hz
∴ Angular velocity, ω = 2πn = 2π × 2 = 4π rad/s
Therefore, moment of inertia of the disc about its own axis,

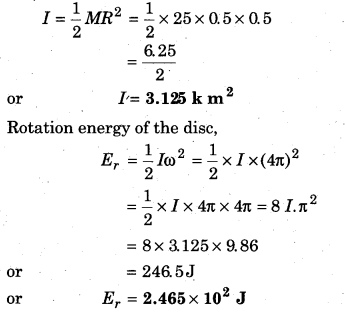
Question 8.
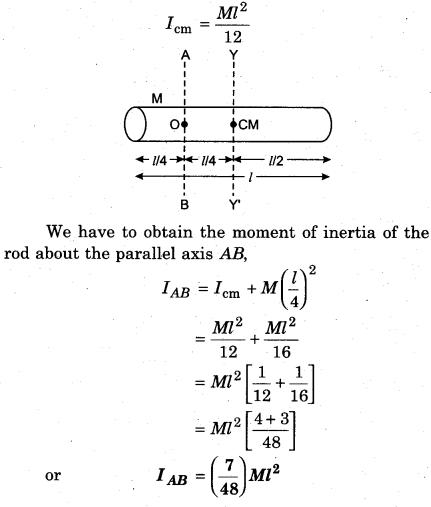
What will be the moment of inertia of a thin rod of mass M and length l along the axis perpendicular to its length and passing through one point from a distance l/4 from one end?
Solution:
The moment of inertia of the rod about the axis passing through its center of mass i.e., about YY’,

Question 9.
Calculate the angular momentum of the spherical Earth rotating along its axis. (Mass of the Earth = 6 × 10²⁴ kg and radius = 6.4 × 10⁶ m)
Solution:
Given Mass of the Earth, M = 6 × 10²⁴ kg;
Radius of Earth, R = 6.4 × 10⁶ m;
Time period of rotation of the Earth, T = 24 hrs. = 24 × 3600 s.
Therefore, moment of inertia of the Earth;

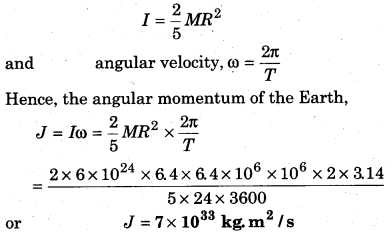
Question 10.
The mass of a hollow sphere is 1 kg and its inner and outer radius are 0.1 m and 0.2 m respectively. Calculate the moment of inertia of the sphere along the diameter and radius of gyration.
Solution:
Given M = 1 kg; outer radius R₁ = 0.2 m; inner radius, R₂ = 0.1m; I = ?; K = ?
Moment of inertia of the hollow sphere about its diameter,

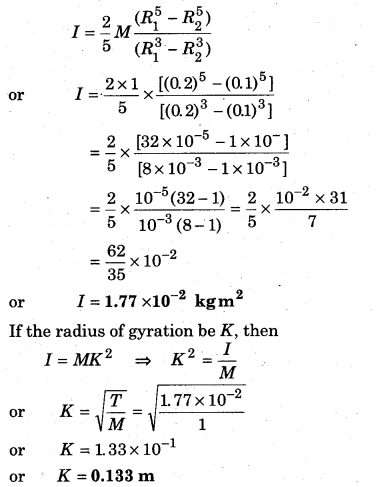
Question 11.
A 1 kg mass ball is moving on a horizontal surface with a velocity 20 m/s and reaches a plane which makes an angle of 30° with the horizontal. If friction is negligible then how much distance vertically will the ball reach?
Solution:
Given;
M = 1kg; u = 20 m/s; θ = 30°

Therefore the retardation of the ball on the inclined plane,

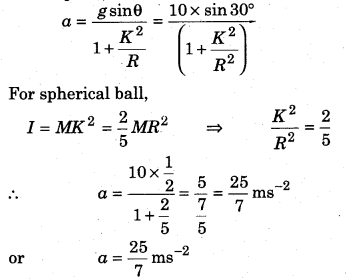
If the ball moves for a distance S along the inclined plane before it stops, then using the relation.
v² = u² + 2as, we have,

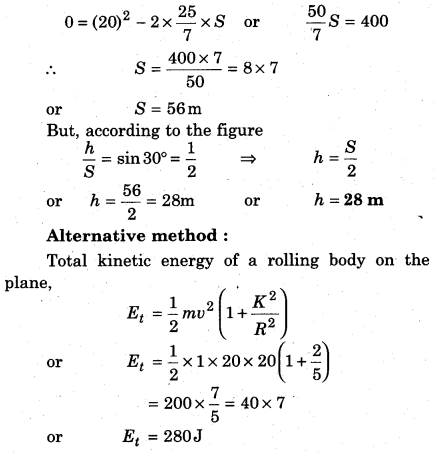
At the top of the inclined plane, the kinetic energy of the moving body is completely converted into the potential energy.
∴ U = E_t
or mgh = E_t
or 1 × 10 × h = 280
⇒ h = 28 m

Question 12.
Three bodies a ring, a solid cylinder and a sphere performing rolling motion on an inclined plane. They start from rest. All the bodies have same radii. Which body will reach the base of the inclined plane with highest velocity?
Solution:
The velocity obtained at the base of an inclined plane by any rolling body,

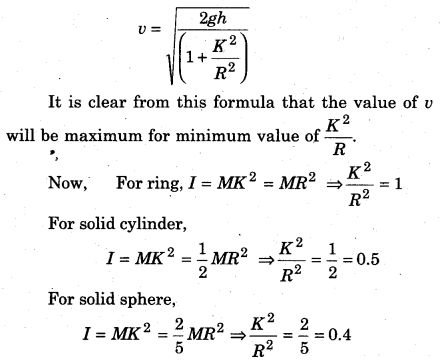
∵ The value of \(\frac{K^{2}}{R}\) is minimum for solid sphere
Therefore the velocity of solid sphere will be maximum.

Question 13.
If keeping the mass constant a rotating disc’s radius is suddenly reduced to half. Then how much would be its new angular velocity value?
Solution:
Initial moment of inertia of the disc,
I₁ = \(\frac{1}{2}\) MR₁²
and initial angular velocity = ω₁
After reducing the radius to half (i.e. ,R₂ = R₁/ 2), the new moment of inertia,
\(I_{2}=\frac{1}{2} M R_{2}^{2}=\frac{1}{2} M \cdot\left(\frac{R_{1}}{2}\right)^{2}=\frac{1}{2} M \frac{R_{1}^{2}}{4}\)
or I₂ = \(\frac{1}{4}\) I₁
If ω₂ be the new angular velocity, then according to the law of conservation of angular momentum,
I₂ ω₂ = I₁ ω₁ or \(\frac{I_{1}}{4}\) ω₂ = I₁ ω₁
or ω₂ = 4ω₁
Thus the angular velocity will become four times.

Question 14.
A body’s angular velocity is changed from 1 cycle/second to 16 cycles/second without applying any torque on it. In both the cases what would be the ratio of radii of gyration?
Solution:
Initial moment of inertia = I₁ and initial angular velocity ω = 2πn₁ = 2π × 1 = 2π rad/s
In second situation, the moment of inertia = I₁ and angular velocity, ω₂ = 2πn₂ = 2π × 16 = 32π rad/s
As no external torque is applied, so according to the law of conservation of angular momentum,

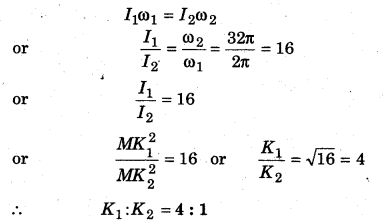
Question 15.
M and N two wheels are on the same axis. The moment of inertia of M is 6 kg m² and is rotating with 600 cycles/min and N is at rest. Joining both of them together by a clutch they do motion by 400 cycles/min. Calculate the moment of inertia of N.
Solution:
Moment of inertia of wheel M,
I₁ = 6kg m²
Frequency of rotation,
n₁ = 600 cycles/min.
= \(\frac{600}{60}\) c.s^-1 = 10 c.s^-1
∴ Initial angular velocity,
ω₁ = 2πn₁ = 2π × 10 = 20n rad/s
Angular velocity of joint wheels,
ω₂ = 2πn₂
where, n₂ = 400 c/min = \(\frac{400}{60}\) c/s = \(\frac{20}{3}\) c/ s
∴ ω₂ = 2π × \(\frac{20}{3}\) = \(\frac{40}{3}\)π rad/s 3 3
If the joint moment of inertia be I₂, then by the law of conservation of angular momentum,
I₂ω₂ = I₁ω₁
or I₂ × \(\frac{40}{3} \pi\) = 6020π
or I₂ = 9kgm²
but I₂ = I_M + I_N
∴ 9 = 6 + I_N
or I_N = 9 – 6 = 3kg m².

RBSE Solutions for Class 11 Physics