RBSE Solutions for Class 12 Maths Chapter 8 Application of Derivatives Ex 8.6

Rajasthan Board RBSE Class 12 Maths Chapter 8 Application of Derivatives Ex 8.6

Question 1.
Show that the triangle of maximum area that can be inscribed in a circle is an equilateral triangle.
Solution:
Let ∆ABC is inscribed in a circle of radius r. A perpendicular E is drawn of AC from the vertex B of triangle which meets AC at D and circle at E.
Let base of triangle AC = 2x
and height of triangle BD = y




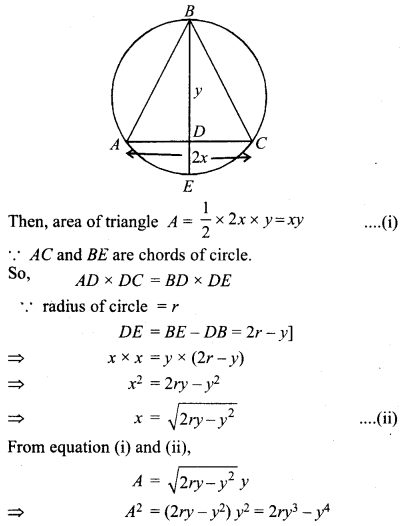
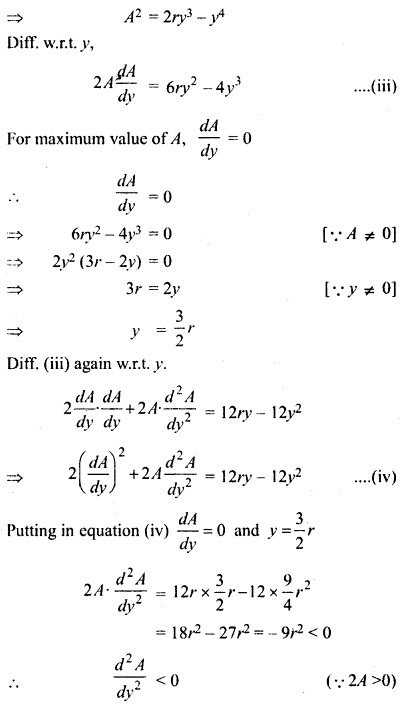
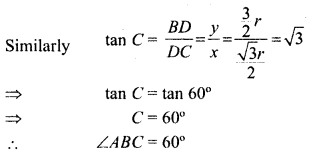
Hence, ∆ABC is an equilateral triangle.

Question 2.
Given the sum of the perimeters of a square and a circle, then prove that the sum of their area is least when one side of the square is equal to diameter of the circle.
Solution:
Let side of square is x and radius of circle is r.
Perimeter of circle (circumference) = 2πr
Sum of both perimeters = 4x + 2πr = k …..(i)
Area of circle, A₂ = πr²
Area of square, A₂ = x²
∴ Sum of areas, A = πr² + x²


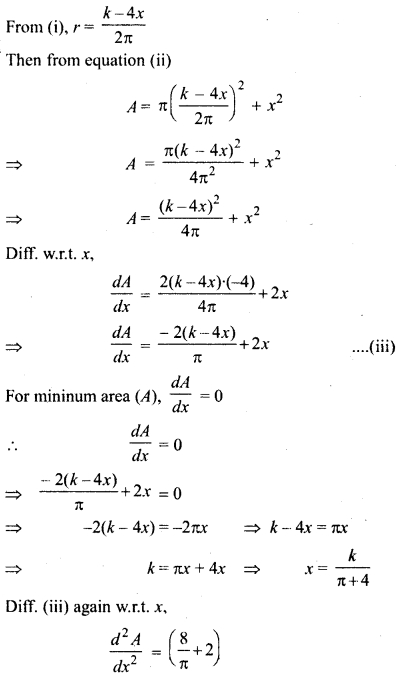
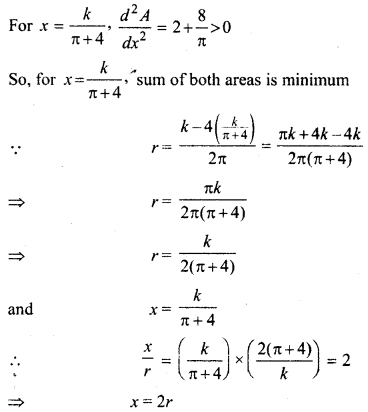
Hence, side of square is equal to diameter of circle, when sum of area of both is minimum.

Question 3.
Show that the cone of the greatest volume which can be inscribed in a given sphere has an altitude equal to \(\frac { 2 }{ 3 } \) of the diameter of the sphere.
Solution:
Let radius of sphere = r
height of cone = x
radius of base of cone = y

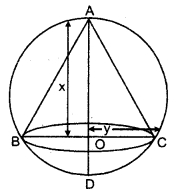
Diameter of sphere and axis of cone will coincide with each other that by maximum height can be taken.


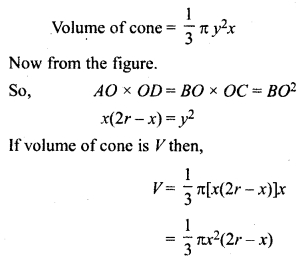
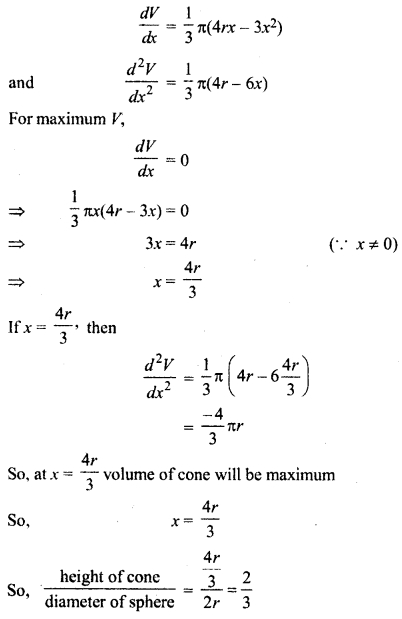
= 2 : 3 or height of cone is \(\frac { 2 }{ 3 } \) of diameter of the sphere.

Question 4.
The expenses per hour for plying steamer in a river is proportionate to cube of its speed. If the speed of the water current is x kin per hour, then prove that the maximum normal (prudent) speed of the steamer will be \(\frac { 2 }{ 3 } \) km per hour if it flied against the current of the water.
Solution:
Let velocity of steamer is u km/h and distance covered is S km.
According to question
Speed of water current = x km/h
and relative velocity of steamer = (u – x) k/h time take to cover the distance
T = \(\frac { S }{ u-x } \) hour
Given expense per hour = Ku³ {K is constant}
Total expenses E = Ku³ . \(\frac { S }{ u-x } \)

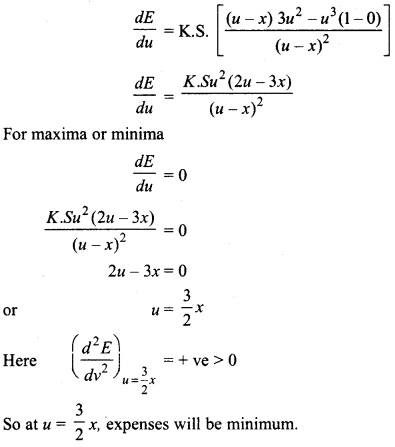
Question 5.
If sum of hypotenuse and one side of right angled triangle is given, then area of triangle is maximum if angle between these sides is \(\frac { \pi }{ 3 } \)
Solution:
Let, in right angle triangle, hypotenuse is h and base is x, angle between them is θ.




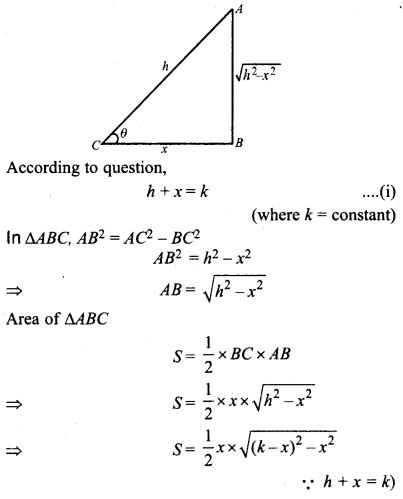
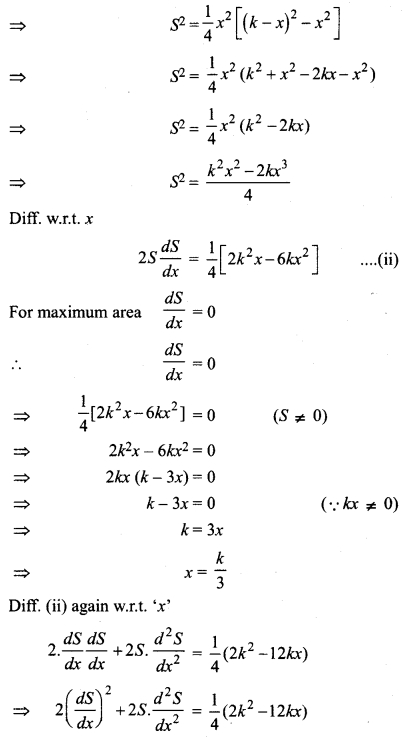
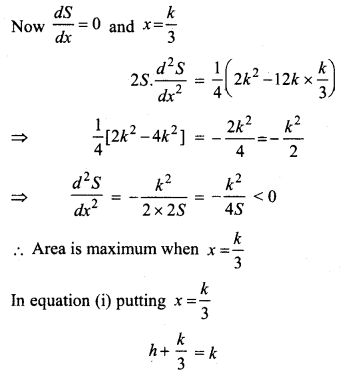
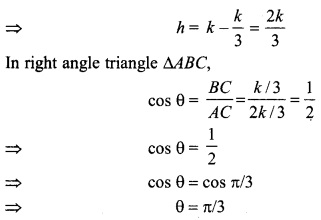
Hence, Area is maximum when θ = π/3

Question 6.
If a circle of radius ‘a’ is inside an equilateral triangle, then prove that minimum perimeter of triangle will be 6\(\sqrt { 3 }\)a.
Solution:
Let ∆ABC is an equilateral triangle.
In which AB = AC
Then, ∠ABC = ∠ACB = 2θ (Let)

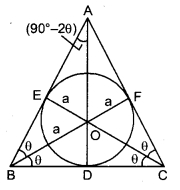
In ∆OBD,
BD = a cot θ
Similarly CD = BE = CF = a cot θ
In ∆AOE,
AE = a cot (90° – 2θ)
= a tan 2θ = AF
AB = AE +BE
= a tan 2θ + a cot θ
BC = BD +CD
= a cot θ + a cot θ
= 2a cot θ
CA = AF + CF
= a tan 2θ + a cot θ
Let perimeter of ∆ABC is P, then
P = AB + BC + CA
= (a tan 2θ + a cot θ) + 2a cot θ + a tan 2θ + a cot θ)
= 2a tan 2θ + 4a cot θ
= 2a(tan 2θ + 2 cot θ) …..(i)
Diff w.r.t. 0,
\(\frac { dp }{ d\theta } \) = 2a (2 sec² 2θ – 2 cosec² θ)
For maxima and minima \(\frac { dp }{ d\theta } \) = 0
⇒ 2a(2 sec² 2θ – 2 cosec² θ) = 0
⇒ sec² 2θ – cosec² θ = 0
⇒ sec 2θ = cosec θ
⇒ cot 2θ = sin θ

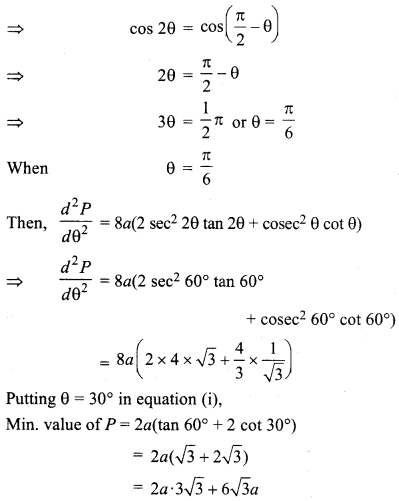
Hence, required minimum perimeter of isosceles triangle = 6\(\sqrt { 3 }\)a

Question 7.
If a normal is drawn a point ‘P’ of ellipse

then Prove that the maximum distance from centre of ellipse will be a – b.
Solution:
We have to show that maximum value of OM is a – b. Now equation of normal at point P (a cos θ, b sin θ), ax sec θ – by cosec θ = a² – b²

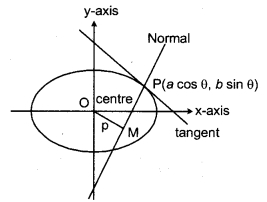
Perpendicular distance between normal and centre of ellipse,

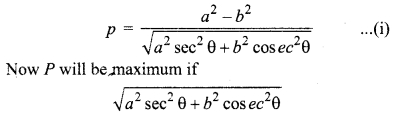
will be minimum.
Here (a² – b²) is constant.
Now, Let y = a² sec² θ + b² cosec² θ
Note : Here, we will prove that (a + b)² is minimum value of a² sec² θ + b² cosec² θ
\(\frac { dy }{ dx } \) = 2a² sec² θ tan θ – 2b² cosec² θ cot θ using \(\frac { dy }{ dx } \) = 0
⇒ 2[a² sec² θ tan θ – b² cosec² θ cot θ] = 0

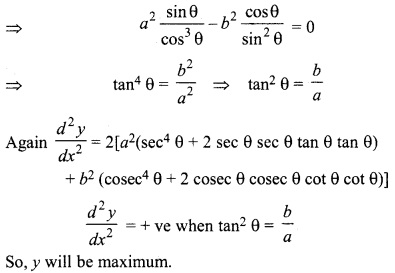
Putting tan² θ = \(\frac { b }{ a } \) in y = a² sec² θ + b² cosec² θ
y = a² sec² θ + b² cosec² θ
= a² (1 + tan² θ) + b² (1 + cot² θ)
= a² [1 + \(\frac { b }{ a } \)] + b² [1 + \(\frac { a }{ b } \)]
= a² + b² + 2ab
= (a + b)²
So, y = a² sec² θ + b² cosec² θ has minimum value (a + b)²
From equation (i),
Maximum value of p

Hence, maximum distance between normal and centre of ellipse is (a – b)

RBSE Solutions for Class 12 Maths